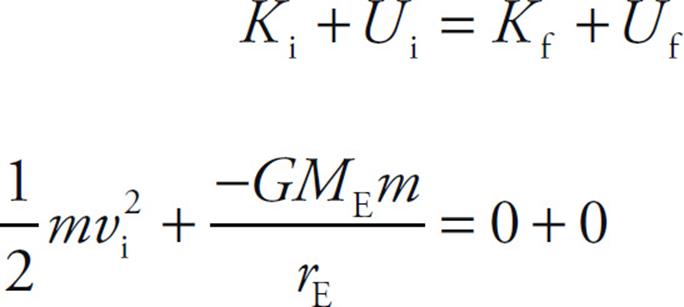
Ap Physics Frq Satellite Of Mass M Orbits Earth Mechanical Energy
AP Physics C Exam
AP® PHYSICS C: MECHANICS 2007 SCORING COMMENTARY Question 2 Overview This question evaluated students’ understanding of orbital motion and energetics. The specific system was an artificial satellite of the planet Mars. The orbital period and the satellite’s orbital speed were given, as were the satellite’s mass and Mars’s radius.
KEPLER’S LAWS
Johannes Kepler spent years of exhaustive study distilling volumes of data collected by his mentor, Tycho Brahe, into three simple laws that describe the motion of planets.
Kepler’s First Law
Every planet moves in an elliptical orbit, with the Sun at one focus.
Kepler’s Second Law
As a planet moves in its orbit, a line drawn from the Sun to the planet sweeps out equal areas in equal time intervals.
Kepler’s Third Law
If T is the period and a is the length of the semimajor axis of a planet’s orbit, then the ratio T2/a3 is the same for all planets orbiting the same star.
NEWTON’S LAW OF GRAVITATION
Newton eventually proved that Kepler’s first two laws imply a law of gravitation: Any two objects in the universe exert an attractive force on each other—called the gravitational force—whose strength is proportional to the product of the objects’ masses and inversely proportional to the square of the center-to-center distance between them. If we let G be the universal gravitational constant, then the strength of the gravitational force is given by the equation:
Consider a mass, m1, close to the surface of Earth. We will use Newton’s Law of Gravitation and Newton’s Second Law to show that the gravitational acceleration of m1 is independent of the mass of the object, as shown below.
The forces F1-on-2 and F2-on-1 act along the line that joins the bodies and form an action/reaction pair.
The first reasonably accurate numerical value for G was determined by Cavendish more than one hundred years after Newton’s Law was published. To three decimal places, the currently accepted value of G is
G = 6.67 × 10–11 N • m2/kg2
Kepler’s Third Law then follows from Newton’s Law of Gravitation. We’ll show how this works, for the case of a circular orbit of radius R (which can be considered an elliptical orbit with eccentricity zero). If the orbit is circular, then Kepler’s Second Law says that the planet’s orbit speed, v, must be constant. Therefore, the planet executes uniform circular motion, and centripetal force is provided by the gravitational attraction of the Sun. If we let M be the mass of the Sun and m be the mass of the planet, then this last statement can be expressed mathematically as:
Note that this formula
makes sense when we
consider that each object
is contributing an
m/r term to the total
force of gravity.
The period of a planet’s orbit is the time it requires to make one revolution around the Sun, so dividing the distance covered, 2πR, by the planet’s orbit speed, v, we have
Equation (1) implies that v2 = GM/R. Squaring both sides of Equation (2) and then substituting v2 = GM/R, we find that
Therefore,
which is Kepler’s Third Law for a circular orbit of radius R.
Acceleration of Gravity Due to Large Bodies
We have been using the acceleration due to gravity g = 10 m/s2 for all objects falling near the surface of Earth. We have assumed that the mass does not affect the acceleration of gravity and now we will show why.
Assume a small mass m is located near a large body (i.e., a planet or star) of mass M. The gravitational force on the object near the surface will equal the mass of the object times the acceleration of gravity ag. The equation below shows how the mass of the object cancels out, and the acceleration of gravity is independent of that mass of the object.
From this expression we can see that the acceleration due to gravity for any object on a planet would be related to the mass and radius of the planet, but not the mass of the object.
THE GRAVITATIONAL ATTRACTION DUE TO AN EXTENDED BODY
Newton’s Law of Gravitation is really a statement about the force between two point particles: objects that are very small in comparison to the distance between them. Newton also proved that a uniform sphere attracts another body as if all of the sphere’s mass were concentrated at its center.
For this reason, we can apply Newton’s Law of Gravitation to extended bodies, that is, to objects that are not small relative to the distance between them.
Additionally, a uniform shell of mass does not exert a gravitational force on a particle inside it. This means that if a spherical planet is uniform, then as we descend into it, only the mass of the sphere underneath us exerts a gravitational force; the shell above exerts no force because we’re inside it.
Example 1 What is the gravitational force on a particle of mass m at a distance x from the center of a spherically symmetric planet of uniform density ρ, total mass M, and radius R for
(a) x ≥ R
(b) x< R
Solution.
(a) If x ≥ R, then the planet can be treated as a point particle with all its mass concentrated at its center, and
(b) However, if x< R, then only the mass within the sphere of radius x exerts a gravitational force on the particle. Since the volume of such a sphere is (4/3)πx3, its mass is (4/3)πx3ρ; we’ll denote this by Mwithinx. Since the mass of the entire planet is (4/3)πR3ρ, we see that
Therefore, the force that this much mass exerts on the particle of mass m is
In summary then,
Example 2 Given that the radius of Earth is 6.37 × 106 m, determine the mass of Earth.
Solution. Consider a small object of mass m near the surface of Earth (mass M). Its weight is mg, but its weight is just the gravitational force it feels due to Earth, which is GMm/R2. Therefore,
Since we know that g = 10 m/s2 and G = 6.67 × 10–11 N•m2/kg2, we can substitute to find
Example 3 We can derive the expression GM/R2 by equating mg and GMm/R2 (as we did in the previous example), and this gives the magnitude of the absolute gravitational acceleration, a quantity that’s sometimes denoted g0. The notation g is acceleration, but with the spinning of Earth taken into account. Show that if an object is at the equator, its measured weight (the weight that a scale would measure), mg, is less than its true weight, mg0, and compute the weight difference for a person of mass m = 60 kg.
Solution. Imagine looking down at Earth from above the North Pole.
The net force toward the center of Earth is F0 – FN, which provides the centripetal force on the object. Therefore,
Since v = 2πR/T, where T is Earth’s rotation period we have
or, since F0 = mg0 and FN = mg,
Since the quantity 4p2mR/T2 is positive, mg must be less than mg0. The difference between mg0 and mg, for a person of mass m = 60 kg, is only:
and the difference between g0 and g is
Example 4 Communications satellites are often parked in geosynchronous orbits above Earth’s surface. Such satellites have orbit periods that are equal to Earth’s rotation period, so they remain above the same position on Earth’s surface. Determine the altitude and the speed that a satellite must have to be in a geosynchronous orbit above a fixed point on Earth’s equator. (The mass of Earth is 5.98 × 1024 kg.)
Solution. Let m be the mass of the satellite, M be the mass of Earth, and R be the distance from the center of Earth to the position of the satellite. The gravitational pull of Earth provides the centripetal force on the satellite, so
The orbit speed of the satellite is 2πR/T, so
which implies that
Now, the key feature of a geosynchronous orbit is that its period matches Earth’s rotation period, T = 24 hr. Substituting the numerical values of G, M, and T into this expression, we find that
Therefore, if rE is the radius of Earth, then the satellite’s altitude above Earth’s surface must be
h = R – rE = (4.23 × 107 m) – (6.37 × 106 m) = 3.59 × 107 m
which is equal to 5.6rE. The speed of the satellite in this orbit is, from the first equation in our calculations,
regardless of the mass of the satellite (well, as long as m< < M).
Example 5 A uniform, slender bar of mass M has length L. Determine the gravitational force it exerts on the point particle of mass m shown below:
Solution. Since the bar is an extended body (and not spherically symmetric), we must calculate F using an integral. Select an arbitrary segment of length dx and mass dM in the bar, at a distance x from its left-hand end.
Then, since the bar is uniform, dM = (M/L)dx, so the gravitational force between m and dM is
Now, by adding (that is, by integrating) all of the contributions dF, we get the total gravitational force, F:
GRAVITATIONAL POTENTIAL ENERGY
When we developed the equation U = mgh for the gravitational potential energy of an object of mass m at height h above the surface of Earth, we took the surface of Earth to be our U = 0 reference level and assumed that the height, h, was small compared to Earth’s radius. In that case, the variation in g was negligible, so g was treated as constant. The work done by gravity as an object was raised to height h was then simply –Fgrav × s = –mgh, so Ugrav, which by definition equals –Wby grav, was mgh.
But now we’ll take variations in g into account and develop a general equation for gravitational potential energy, one that isn’t restricted to small altitude changes.
Consider an object of mass m at a distance r1 from the center of Earth (or any spherical body) moving by some means to a position r2:
How much work did the gravitational force perform during this displacement? The answer is given by the equation:
Therefore, since Ugrav = –Wby grav, we get
Let’s choose our U = 0 reference at infinity. That is, we decide to allow U2 → 0 as r2 → ∞. Then this equation becomes
Notice that, according to this equation (and our choice of U = 0 when r = ∞), the gravitational potential energy is always negative. This just means that energy has to be added to bring an object (mass m) bound to the gravitational field of M to a point very far from M, at which U = 0.
A PROOF OF EQUATION (*)
Because the gravitational force is not constant over the displacement, the work done by this force must be calculated using a definite integral:
Displacement can be broken into a series of infinitesimal steps of two types: Those that are at a constant distance from Earth’s center and those that are along a radial line going away from Earth’s center. The result is that the displacement is equivalent to the sum of two curves C1 + C2:
The gravitational force along C1 does no work, because it’s always perpendicular to the displacement. Along C2, the inward gravitational force is in the opposite direction from the outward displacement, so along this ray,
Therefore,
Example 6 With what minimum speed must an object of mass m be launched in order to escape Earth’s gravitational field? (This is called escape speed, vesc.)
Solution. When launched, the object is at the surface of Earth (ri = rE) and has an upward, initial velocity of magnitude vi. To get it far away from Earth, we want to bring its gravitational potential energy to zero, but to find the minimum launch speed, we want the object’s final speed to be zero by the time it gets to this distant location. So, by Conservation of Energy,
Note: This is the minimum
speed needed to escape
Earth’s gravitational field
but, in this situation, a
projectile would also have
to contend with the Sun’s
gravitational field.
which gives
Substituting the known numerical values for G, ME, and rE gives us:
Example 7 A satellite of mass m is in a circular orbit of radius R around Earth (radius rE, mass M).
(a) What is its total mechanical energy (where Ugrav is considered zero as R approaches infinity)?
(b) How much work would be required to move the satellite into a new orbit, with radius 2R?
Solution.
(a) The mechanical energy, E, is the sum of the kinetic energy, K, and potential energy, U. You can calculate the kinetic energy since you know that the centripetal force on the satellite is provided by the gravitational attraction of Earth:
Therefore,
(b) From the equation Ki + Ui + W = Kf + Uf, we see that
W = (Kf + Uf) − (Ki + Ui)
= Ef − Ei
Therefore, the amount of work necessary to effect the change in the satellite’s orbit radius from R to 2R is
A Note on Elliptical Orbits
The expression for the total energy of a satellite in a circular orbit of radius R [derived in Example 7(a)] is:
And this also holds for a satellite traveling in an elliptical orbit, if the radius R is replaced by a, (the length of the semimajor axis):
Example 8 An asteroid of mass m is in an elliptical orbit around the Sun (mass M). Assume that m<< M.
(a) What is the total energy of the asteroid?
(b) What is the ratio of v1 (the asteroid’s speed at aphelion) to v2 (the asteroid’s speed at perihelion)? Write your answer in terms of r1 and r2.
(c) What is the time necessary for the asteroid to make a complete orbit around the Sun?
Solution.
(a) The total energy of the asteroid is equal to –GMm/2a, where a is the semimajor axis. However, notice in the figure above that 2a = r1 + r2. Therefore, the total energy of the asteroid is
(b) One way to answer this question is to invoke Conservation of Angular Momentum. When the asteroid is at aphelion, its angular momentum (with respect to the center of the Sun) is L1 = r1mv1. When the asteroid is at perihelion, its angular momentum is L2 = r2mv2. Therefore,
This tells us that the asteroid’s speed at aphelion is less than its speed at perihelion (because r2/r1< 1), as implied by Kepler’s Second Law (a line drawn from the Sun to the asteroid must sweep out equal areas in equal time intervals). The closer the asteroid is to the Sun, the faster it has to travel to make this true.
(c) As you know, the time necessary for the asteroid to make a complete orbit around the Sun is the orbit period, T. Using Kepler’s Third Law, with a = (r1 + r2), we find
ORBITS OF THE PLANETS
Kepler’s First Law states that the planets’ orbits are ellipses, but the ellipses that the planets in our solar system travel are nearly circular. The deviation of an ellipse from a perfect circle is measured by a parameter called its eccentricity. The eccentricity, e, is the ratio of c (the distance between the center and either focus) to a, the length of the semimajor axis. For every point on the ellipse, the sum of the distances to the foci (plural of focus) is a constant (and is equal to 2a in the figure below).
Kepler’s First Law also states that one of the foci of a planet’s elliptical orbit is located at the position of the Sun. Actually, the focus is at the center of mass of the Sun-planet system, because when one body orbits another, both bodies orbit around their center of mass, a point called thebarycenter.
For most of the planets, which are much less massive than the Sun, this correction to Kepler’s First Law has little significance, because the center of mass of the Sun and the planet system is close enough to the Sun’s center. For example, let’s figure out the center of mass of the Sun-Earth system. The mass of Earth is m = 5.98 × 1024 kg, the mass of the Sun is M = 1.99 × 1030 kg, and the Sun-Earth distance averages R = 1.496 × 1011 m. Therefore, letting x = 0 be at the Sun’s center, we have
So the center of mass of the Sun-Earth system is only 450 km from the center of the Sun, a distance of less than 0.1% of the Sun’s radius.
Example 9 Derive a corrected version of Kepler’s Third Law for the following orbiting system. Both bodies have orbit period T.
Solution. The centripetal force on each body is provided by the gravitational pull of the other body, so
which imply
But, since both bodies have the same orbit period, T, we have
Substituting these results into the preceding pair of equations gives us:
which simplify to
Adding this last pair of equations gives us the desired result:
Note that this final equation is a general version of Kepler’s Third Law for a circular orbit derived earlier, T2/R3 = 4π2/GM, where it was assumed that the planet orbited at a distance R from the center of the Sun.
Example 10 An artificial satellite of mass m travels at a constant speed in a circular orbit of radius R around Earth (mass M). What is the speed of the satellite?
Solution. The centripetal force on the satellite is provided by Earth’s gravitational pull. Therefore,
Solving this equation for v yields
Notice that the satellite’s speed doesn’t depend on its mass; even if it were a baseball, if its orbit radius were R, then its orbit speed would still be .
CHAPTER 9 REVIEW QUESTIONS
The answers and explanations can be found in Chapter 16.
Section I: Multiple Choice
1. If the distance between two point particles is doubled, then the gravitational force between them
(A) decreases by a factor of 4
(B) decreases by a factor of 2
(C) increases by a factor of 2
(D) increases by a factor of 4
(E) cannot be determined without knowing the masses
2. At the surface of Earth, an object of mass m has weight w. If this object is transported to a height above the surface that’s twice the radius of Earth, then, at the new location,
(A) its mass is m/2 and its weight is w/2
(B) its mass is m and its weight is w/2
(C) its mass is m/2 and its weight is w/4
(D) its mass is m and its weight is w/4
(E) its mass is m and its weight is w/9
3. A moon of mass m orbits a planet of mass 100m. Let the strength of the gravitational force exerted by the planet on the moon be denoted by F1, and let the strength of the gravitational force exerted by the moon on the planet be F2. Which of the following is true?
(A) F1 = 100F2
(B) F1 = 10F2
(C) F1 = F2
(D) F2 = 10F1
(E) F2 = 100F1
4. The dwarf planet Pluto has 1/500 the mass and 1/15 the radius of Earth. What is the value of g (in m/s2) on the surface of Pluto?
(A)
(B)
(C)
(D)
(E)
5. A satellite is currently orbiting Earth in a circular orbit of radius R; its kinetic energy is K1. If the satellite is moved and enters a new circular orbit of radius 2R, what will be its kinetic energy?
(A) K1/4
(B) K1/2
(C) K1
(D) 2K1
(E) 4K1
6. A moon of Jupiter has a nearly circular orbit of radius R and an orbit period of T. Which of the following expressions gives the mass of Jupiter?
(A) 2πR/T
(B) 4π2R/T2
(C) 2π R3/(GT2)
(D) 4πR2/(GT2)
(E) 4π2R3/(GT2)
7. Two large bodies, Body A of mass m and Body B of mass 4m, are separated by a distance R. At what distance from Body A, along the line joining the bodies, would the gravitational force on an object be equal to zero? (Ignore the presence of any other bodies.)
(A) R/16
(B) R/8
(C) R/5
(D) R/4
(E) R/3
8. The mean distance from Saturn to the Sun is 9 times greater than the mean distance from Earth to the Sun. How long is a Saturn year?
(A) 18 Earth years
(B) 27 Earth years
(C) 81 Earth years
(D) 243 Earth years
(E) 729 Earth years
9. The Moon has mass M and radius R. A small object is dropped from a distance of 3R from the Moon’s center. The object’s impact speed when it strikes the surface of the Moon is equal to for k =
(A) 1/3
(B) 2/3
(C) 3/4
(D) 4/3
(E) 3/2
10. Two satellites, A and B, orbit a planet in circular orbits having radii RA and RB, respectively, as shown above. If RB = 3RA, the velocities vA and vB of the two satellites are related by which of the following?
(A) vB = vA
(B) vB = 3vA
(C) vB = 9vA
(D) vB = vA
(E) vB =
Section II: Free Response
1. Consider two uniform spherical bodies in deep space. Sphere 1 has mass m1 and Sphere 2 has mass m2. Starting from rest from a distance R apart, they are gravitationally attracted to each other.
(a) Compute the acceleration of Sphere 1 when the spheres are a distance R/2 apart.
(b) Compute the acceleration of Sphere 2 when the spheres are a distance R/2 apart.
(c) Compute the speed of Sphere 1 when the spheres are a distance R/2 apart.
(d) Compute the speed of Sphere 2 when the spheres are a distance R/2 apart.
Now assume that these spheres orbit their center of mass with the same orbit period, T.
(e) Determine the radii of their orbits. Write your answer in terms of m1, m2, T, and fundamental constants.
2. A satellite of mass m is in the elliptical orbit shown below around Earth (radius rE, mass M). Assume that m<< M.
(a) Determine v1, the speed of the satellite at perigee (the point of the orbit closest to Earth). Write your answer in terms of r1, r2, M, and G.
(b) Determine v2, the speed of the satellite at apogee (the point of the orbit farthest from Earth). Write your answer in terms of r1, r2, M, and G.
(c) Express the ratio v1/v2 in simplest terms.
(d) What is the satellite’s angular momentum (with respect to Earth’s center) when it’s at apogee?
(e) Determine the speed of the satellite when it’s at the point marked X in the figure.
(f) Determine the period of the satellite’s orbit. Write your answer in terms of r1, r2, M, and fundamental constants.
Summary
Newton’s Law of Gravitation
- Newton’s Law of Gravitation gives the force between any two point masses, regardless of their mass or location. This uniform circular motion can also be described by an angular velocity and a centripetal acceleration.
Circular Orbits
General Orbits
- The gravitational potential energy is represented by the following formula:
- Both mechanical energy and angular momentum are conserved for orbits.
Etotal = 0
- Systems are bound when the total mechanical energy is less than zero. This means that the gravitational potential energy is greater than the kinetic energy of the system.
- For an object to escape, its kinetic energy must be greater than or equal to its gravitational potential energy.
for M1 to escape the pull of M2.
Gravity of Spheres and Shells
- The gravity due to a spherical shell of mass, M, and radius, R, where the mass is at a distance, r, away from the center of the shell is represented by the equations below.
Outside the shell is
and the gravity inside the shell is Fg = 0
- The gravity due to a uniform, solid sphere where a small mass, m, is at a distance, r, away from the center of the sphere is represented by the equations below.
Outside the sphere is
and the gravity inside the shell is
- The gravity of a spherically symmetric sphere of mass, M, and radius, R (that is, ρ(r)), is represented by the equation
A. Kinematics (The Study of Describing Motion)
video A1: Distance, Position, Displacement, Speed, Velocity and Acceleration. Quickly explains what is meant by each of these terms including average and instantaneous velocity.
video A3: A Quick and Intuitive Way to Solve Some Kinematics Problems. This video uses the idea of average velocity to quickly solve some types of kinematics problems. It is a good method for quickly checking your answers on kinematics tests.
video A4: A Quick and Intuitive Way to Solve Some Free Fall Problems. This video uses the idea of average velocity to quickly solve some types of kinematics problems. It is a good method for quickly checking your answers on kinematics tests.
video A5: A Quick and Intuitive Way to Solve Some Projectile Motion Problems. This video uses the idea of average velocity to quickly solve some types of kinematics problems. It is a good method for quickly checking your answers on kinematics tests.
video A6: Projectile Motion Basics.Just the basics of projectile motion.
video A7: Relative Velocity. All velocities are relative, this video describes how to find the velocity of one object relative to another object using vectors.
video A8: Vector Basics (Part I). Just the basics of vector notation including an explanation of unit vectors.
video A9: Vector Basics (part 2)Vector Basics (part 2). Some basics of the relationship between velocity and acceleration vectors.B. Projectile Motion
B. The Big Picture
video B1: The Big Picture (part 1): The Big Picture. This video develops a concept map that links the various topics covered in mechanics. It is just an overview of the material that will be covered in mechanics. Each of these topics will be covered in much more detail later.
video B2 The Big Picture (part 2): More summarizing of the big picture linking kinematics, newton's laws, energy, and power. Again, more detail for each topic will be covered at a later time.
video B3: The Gravitational Force. This video gives a few different viewpoints of the gravitational force and described by Newton's Law of Universal Gravity.
video B4: Gravitational Potential Energy. This video gives a few different viewpoints of gravitational potential energy. More information on gravitational potential energy will be given in units D and G.
video B5: Gravitational Forces and Energies: This video shows how the universal equations for gravitational force and energy transition into their more simple forms when you are near the earth's surface.
C. Newton’s Laws
video C1: Solving Newton's Second Law Problems. This video shows the steps to solving a Newton's second law problem with particular attention given to an object moving on an inclined plane.
video C2:Inclined Planes (Continued from Video C1): This video is continued from video C1. It looks at an object on an inclined plane where friction is present.
video C3: Solving Problems Involving Multiple-Bodies Using Newton's Laws: This videos explains how to find the acceleration and forces for a problem involving 2 or more bodies.
video C3.1:Atwood's Machine Problems. This video shows a method for solving Newton's second law problems involving pulleys.
video C4:Atood's Machine Problems (part II). This video finishes up the analysis of Atwood's machine problems which are problems involving pulleys.
video C5:Circular Motion Problems. This video shows how Newton's laws are applied to circular motion problems.
video C6:Circular Motion Problems (part 2). This video looks at cars travelling around an unbanked turn.
(new) video C6.5: Circular Motion for Non-Uniform Circular Motion. Explains how Newton's 2nd law is used to analyze an object that is traveling in non-uniform circular motion. (Non-uniform circular motion is circular motion in which the object is either speeding up or slowing down.)
video C7:Banked Turns and Toy Airplanes. This is video details the physics of a car travelling around a circle on a banked turn.
video C8:Elevator Problems and Newton's Laws. This video shows how to handle a typical elevator problem involving Newton's laws of motion.
video C9: Review of Unit C. Newton's Laws (part 1). This video is a review of the concepts covered in unit C. Try pausing the video after each question to see if you can answer the question on your own.
video C10: Review of Unit C (part 2)
video C11: Review of Unit C (part 3)
D. Work, Energy, and Power
video D0: Part 1 of the Dot Product or Scalar Product. I originally forgot to add this one. Watch this before D1.
video D1:The Dot Product or the Scalar Product. This video explains one of two ways to multiply two vector quantities. The second method will be covered in a different video.
video D2: The Integral and Work Done by a Force. This video is an introduction to the work done by a varying force.
video D3: Using Calculus to Derive the Kinematics Equations for Straight-line Motion with Constant Acceleration. This video attempts to do just what it says.
video D4: Work Done on an Object by a Constant Force.
video D5:Work Done on an Object by a Varying Force.
video D6:Power and Efficiency
video D7:Conservative and Non-Conservative Forces. This video details the difference between conservative and non-conservative forces in relation to the work done by each type of force.
video D8:Conservative and Non-Conservative Forces (part 2)
video D9:Work Done By a Conservative Forces. This video details how the work done by a conservative force, such as the gravitational force, will equal the negative change in the system's potential energy.
video D10:Unit D Review (part 1). This video is a review of the concepts covered in unit D. Try pausing the video after each question to see if you can answer the question on your own.
video D11:Unit D Review (part 2)
video D12:Unit D Review (part 3)
video D13:Unit D Review (part 4)
E. Momentum, Impulse, and the Center of Mass
From learning these ideas, one should be well equipped for further exploring into the study of this scale, which is essential in jazz music.In terms of technique, to be able to play the phrases, particularly the wide intervallic ones in full speed that Don demonstrates on the CD, obviously requires good technique, or at least will lead to the development of your alternate/sweep picking technique so as to accommodate some unusual runs within certain phrases. Legendary guitarist and educator Don Mock exposes the closely-guarded “secret” soloing techniques of jazz and rock giants, revealing easy ways to create ultra-cool sounding lines and patterns by substituting simple harmonic minor patterns over dominant 7th chords. These book/CD packages each contain over 60 music examples, lines, licks and patterns. All music is written in standard notation and tablature.This book displays the ideas of how to apply the melodic minor scale, through the means of scalar sequences, static chord vamp, ii-V applications, arpeggio superimpositions, pentatonic fragments, etc. Don mock guitar secrets revealed pdf files.
video E1: Momentum Basics. This video shows how momentum conservation follows from Newton's laws of motion.
video E2:Types of Collisions. This video details the different types of collisions between two objects.
video E3:Types of Collisions (part 2).
video E4:Collisions in 2 Dimensions. This video details the physics that occurs when the collision is not 'head-on' but rather 'off-center' so that the two objects travel off of their original straight-line paths.
video E5:Ballistic Pendulum Problems. This video details the physics of a ballistic pendulum problem. An example of a ballistic pendulum problem would be a block hanging from a string getting hit by a bullet so that the bullet-block system swings up to a particular height before it swings back down to its original position.
video E6: Unit E Review (part 1). This video is a review of the concepts covered in unit E on Momentum and Impulse. Try pausing the video after each question to see if you can answer the question on your own.
video E7: Unit E Review (part 2).
video E8: Unit E Review (part 3).
F. Rotational Motion
video F1:Rotational Kinematics. This video explains rotational kinematics for constant angular acceleration.
video F2: Rotational Kinematics (part 2). This video continues to explain rotational kinematics and details how the linear quantities are related to rotational (or angular) quantities as an object rotates.
video F3:Rotational Kinematics (part 3)
video F4: Torque and the Cross-Product (or Vector Product): This video explains a little bit about torque and the cross-product or vector product of two vectors. So this is the second way that two vector quantities can be multiplied together.
video F5:Torque and the Cross-Product (part 2)
video F6: Torque and the Cross-Product (part 3)
video F7: Static Equilibrium Problems. This is a video that explains the necessary conditions for keeping a structure in static equilibrium. Static equilibrium occurs when a system has no linear acceleration and no angular acceleration.
video F8:Static Equilibrium Problems (part 2)
video F9: Rotational Inertia or Moment of Inertia. This video introduces the concept of rotational inertia (or rotational sluggishness or moment of inertia)
(new) video F9.1:Rotational Inertia for a Long Slender Rod. This video derives the moment of inertia (I) for a long slender rod that has a uniform linear mass density.
(new) video F9.2: Rotational Inertia for a Solid Disk. This video derives the moment of inertia (I) for a solid disk of uniform mass density about an axis that runs through its center and is normal to the plane of the disk.
(new) video F9.3:Rotational Inertia for a Solid Cylinder. This video derives the moment of inertia (I) for a solid cylinder of uniform density with its axis through the center.
(new) video F9.4:Rotational Inertia for a Slender Rod of NON-UNIFORM mass density. This video derives the moment of inertia (I) for a long slender rod of NON-UNIFORM mass density with its axis at the end of the rod and perpendicular to it.
(new) video F9.5:Rotational Inertia for a Solid Cylinder of NON-UNIFORM mass density. This video derives the moment of inertia (I) for a solid cylinder of NON-UNIFORM density with its axis through the center.
video F10: The Parallel-Axis Theorem. If you know a system's rotational inertial about an axis that passes through its center of mass, then you can find the rotational inertial about a second axis that is parallel to the original axis by using the parallel-axis theorem.
video F11:Rotational Dynamics (Newton's Second Law in Rotational Motion)
video F12:Rotational Dynamics (part 2)
video F13:Rotational Dynamics (part 3): This is a video that details Atwood's machine problems where the pulley has significant mass.
video F14: Rotational Kinetic Energy (part 1)
video F15: Rotational Kinetic Energy (part 2)
video F15.1: An example of an Atwood's Machine problem involving a pulley with non-negligible mass and with friction in its axle.
video F16:Angular Momentum
Predator games free download. video F17: Angular Momentum (part 2)
video F18: Unit F Review (part 1). This video is a review of the concepts covered in unit F on Rotational Motion. Try pausing the video after each question to see if you can answer the question on your own.
video F19: Unit F Review (part 2)
G. Satellite Motion
This video gives a few different viewpoints of the gravitational force and described by Newton's Law of Universal Gravity.
This video gives a few different viewpoints of gravitational potential energy. More information on gravitational potential energy will be given in units D and G.
video G3: Gravitational Forces and Energies:
This video shows how the universal equations for gravitational force and energy transition into their more simple forms when you are near the earth's surface.
video G4:The Gravitational Field Strength. This video is on the gravitational field due to a planet.
video G5:Satellites in Circular Orbit. This video details the physics of satellites in circular orbit around another object.
video G6: Kepler's 3 Laws of Planetary Motion.
video G7:Elliptical Orbits and the Conservation of Energy
video G8: Elliptical Orbits and the Conservation of Angular Momentum
Video G9: Escape Velocity. Explains how you calculate an object's escape velocity for a given planet.
video G10: The Physics of Binary Star Systems
video G11: Review for Unit G (part 1). This video is a review of the concepts covered in unit G on Planetary or Satellite Motion. Try pausing the video after each question to see if you can answer the question on your own.
video G12: Review for Unit G (part 2)
video G13: Review for Unit G (part 3)
H. Periodic Motion (Harmonic Motion)
video H1: The Kinematics of Harmonic Motion (part 1): An Introduction into Harmonic Motion. This video compares the motion of an object travelling in uniform circular motion to an object travelling in simple harmonic motion. Kinematics is the study of DESCRIBING motion. Dynamics is the study of the CAUSES of motion.
video H2:The Kinematics of Harmonic Motion (part 2)
video H3: The Kinematics of Harmonic Motion (part 3)
video H4: The Dynamics of Simple Harmonic Motion (part 1). This video explains how Newton's laws apply to simple harmonic motion.
video H5: Energy Conservation and Simple Harmonic Motion
video H6:Harmonic Oscillator with a Crate on Top of a Crate. This video details the physics of a crate that is attached to a spring with another create sitting on top of the first one. The net force on the top crate is supplied only by the force of friction.
video H7: Springs in Series and Parallel. This video explains how to find the equivalent spring constants of either set of springs that are connected in parallel and or connected in series.
video H8: The Period of a Simple Pendulum. This video describes the derivation for the period of a simple pendulum.
video H9: The Period of a Physical Pendulum. This video describes the derivation for the period of a physical pendulum.
video H10: The Period of a Physical Pendulum (part 2). This video shows how to apply the equation derived in video H9.
video H11: The Equivalence of a Vertical Mass on a Spring to a Horizontal Mass on a Spring. This video attempts to explain why the physics of a mass attached to a horizontal spring has many similarites to the physics of a mass attached to a vertical spring.
video H12:Review of Unit H (part 1). This video is a review of the concepts covered in unit H on Harmonic Motion. Try pausing the video after each question to see if you can answer the question on your own.
video H13: Review of Unit H (part 2).
video H14: Review of Unit H (part 3)
video H15: Review Unit H (part 4)
video H 16: Review Unit H (part 5)
N. Growth and Decay in Physics (Air Resistance)*
video N1:Objects Falling With Air Resistance.
video N2:Objects Falling With Air Resistance (part 2)
video N3:Review of Air Resistance
video N4: Review of Air Resistance (part 2). Only a part of this video is air resistance. The other part of this video is on RC circuits.